What is the Missing Justification in the Proof of the Angle Bisector Construction?
Introduction
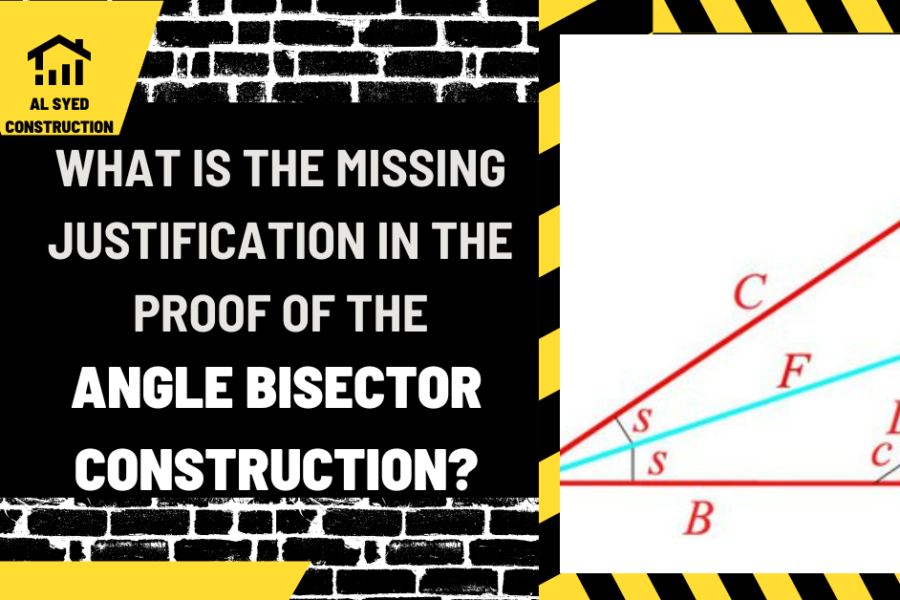
The construction of an angle bisector is a fundamental concept in geometry, involving the division of an angle into two equal parts. While the process of constructing an angle bisector is straightforward, proving its validity requires a clear understanding of geometric principles. This article explores the missing justification often encountered in the proof of the angle bisector construction and its importance in validating the construction.
The Construction of an Angle Bisector
Steps of Construction
The construction of an angle bisector typically involves the following steps:
- Draw the given angle.
- With the vertex of the angle as the center, draw an arc that intersects both sides of the angle.
- Using the points of intersection as centers, draw two arcs of equal radius within the angle, ensuring that they intersect each other.
- Draw a straight line from the vertex of the angle to the point of intersection of the two arcs. This line is the angle bisector.
Geometric Principles Involved
The construction relies on several geometric principles, including the congruence of arcs and the properties of isosceles triangles. These principles ensure that the constructed line indeed bisects the angle.
The Missing Justification
The Role of Congruent Triangles
The missing justification in the proof of the angle bisector construction often relates to the congruence of triangles formed during the process. Specifically, it is necessary to prove that the two triangles formed by the bisector and the sides of the original angle are congruent.
Application of the Side-Angle-Side (SAS) Postulate
The Side-Angle-Side (SAS) postulate can provide the missing justification. By demonstrating that two sides and the included angle of one triangle are congruent to the corresponding parts of the other triangle, we can conclude that the triangles are congruent. This, in turn, confirms that the constructed line bisects the angle.
Implications of the Missing Justification
Ensuring the Validity of the Construction
Providing the missing justification is crucial for ensuring the validity of the angle bisector construction. Without this proof, the accuracy of the construction remains uncertain.
Reinforcing Geometric Understanding
Addressing the missing justification reinforces the understanding of geometric principles and theorems. It highlights the interconnectedness of various concepts in geometry and their application in constructions and proofs.
Conclusion
The missing justification in the proof of the angle bisector construction typically involves proving the congruence of triangles formed during the construction process. By applying the Side-Angle-Side postulate, we can provide the necessary justification, ensuring the validity of the construction and reinforcing geometric understanding. Addressing this missing element is essential for a complete and rigorous proof of the angle bisector construction.